Quantum computation manipulates vector spaces.
A qubit is a 2 dimensional space.
|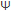 > = a |0>+ ▀ |1> avec |a|2 + |▀|2 = 1
> = a |0>+ ▀ |1> avec |a|2 + |▀|2 = 1
The state space of a composite system is the tensor product of individual spaces.
|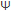 12> = |
12> = |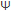 1>
1>![]() |
|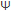 2>
2>
The evolution of a closed system is represented by a unitary matrix.
|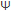 (0)> -> |
(0)> -> |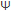 (t)> = U(t,0) |
(t)> = U(t,0) |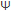 (0)>
(0)>
When we measure a qubit we get one of the eigenvector with a probability equal to the squares of the coefficient magnitude.
|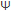 > = a |0>+ ▀ |1> : probability of measuring |0> is |a|2
> = a |0>+ ▀ |1> : probability of measuring |0> is |a|2



 preamble
preamble basis
basis existing representations
existing representations representations
representations